Calculation form 1 for χ2 distribution. Dr.Funatsu, Pofessor of Meisei university, Tokyo. EMail　Contents of forms　Top page

...

[Input] Degree of freedom n=
Input as a≦b. If input b＜a, the values of a and b are exchanged.
E is an index of calculation errors and 0.0001≦E≦0.01
a= b=
E=

[Output]
Value for calculation a=
Value for calculation b=
Value for calculation E=
Probability density(a)=
Probability density(b)=
Mean of χ2=
Variance of χ2=
Mode of χ2=


Area of χ2≦a(probability A)
  =
Area of a＜χ2≦b(probability B)
  =
Area of b＜χ2(probability C)
  =
Total area(A+B+C)=1

Positions of a and b are fixed in the image below, irrespective of input values in the
left column.

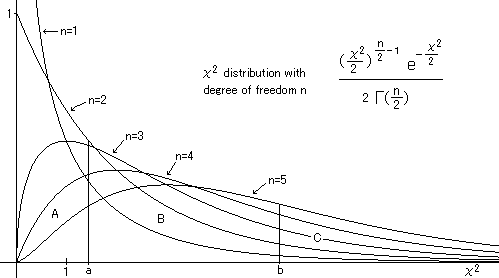
.........................................................................To seek b for given C.

Referring value of gamma distribution, n= (possible errors in decimals of lower
position.)
　Γ(n/2)=

When n is large, values may be shown in index style as 3.14e+12.
When probabilities in the left column, values may be shown as 3.14e-12.
Be careful of last place of decimals.


Comparison of calculated values for area b～∞ of the distribution. Errors vary by E. The smaller E is, the
smaller become the errors. Following tables are of the exsamples of errors.
 
(Decimals not rounded） 
Degree of freedom 1   b=1      b=3       b=5       b=10       b=15        b=20
E=0.01                0.318    0.0830    0.0252    0.001561   0.0001072   0.000007
E=0.001               0.3174   0.08323   0.02535   0.001564   0.0001074   0.000007
E=0.0001              0.3172   0.08326   0.02434   0.001565   0.0001075   0.000007

Degree of freedom 2   b=2      b=5       b=10        b=20               
E=0.01                0.368    0.081     0.00672     0.0000455
E=0.001               0.3677   0.0821    0.006736    0.0000454
E=0.0001              0.3678   0.0820    0.006737    0.0000453

Degree of freedom 5   b=5       b=10       b=20         b=30        
E=0.01                0.4152    0.0750     0.00125      0.00001478
E=0.001               0.4159    0.07522    0.0012499    0.000014719
E=0.0001              0.4158    0.07523    0.0012496    0.000014715